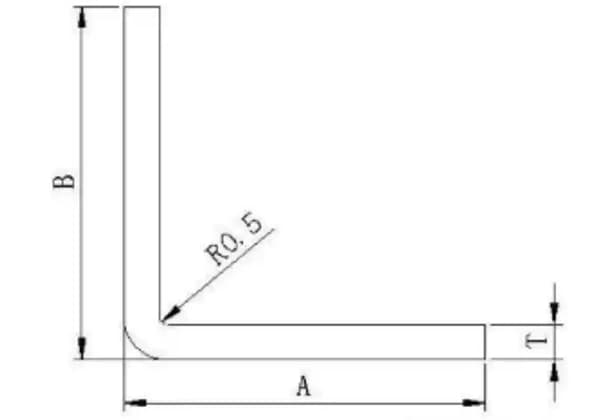
Have you ever wondered why some metal parts spring back after bending? In this article, we’ll explore the fascinating world of bending dies and how to accurately predict springback. You’ll learn practical tips and formulas to achieve precise results in your metalworking projects.
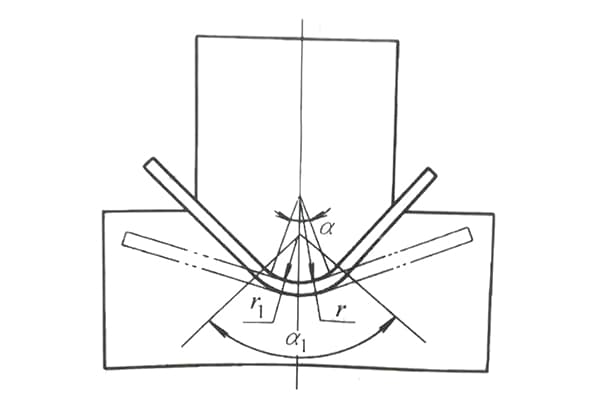
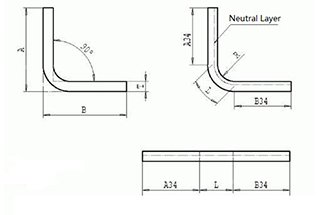
When designing a bending die with an internal arc, many people either choose to use the same R value as the original product and do not consider the springback, or directly reduce the R value by a certain factor.
For example, if the original product has an R value of 1 and the material is relatively hard, they would choose 0.8 times the R value for the convex mold, which would be 0.8.
If the material is relatively soft, they would choose 0.9 times the R value, which would be 0.9.
If there is any deviation, they modify the mold several times based on experience to achieve tolerance within the range.
However, if this method is used to design a product with a thickness of 0.5 and an internal R value of 200mm, it may be difficult to accurately predict the amount of springback.
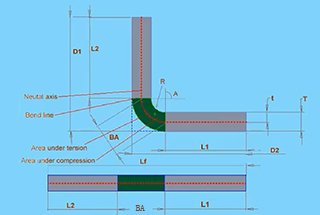
Therefore, a universal formula for springback is introduced below, which can be used to calculate the springback value based on numerical input.
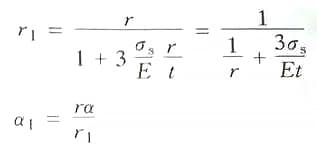
In the formula:
Assuming 3σs/E=A as the simplification coefficient, with values listed in Table 2-27. The calculation formula for the convex die corner radius during the bending of circular section bars is as follows:

The value of A is shown in the table below.
| Material Science | State | A | Material Science | State | A |
| 1035(L4) 8A06(L6) | annealing | 0.0012 | QBe2 | soft | 0.0064 |
| Cold hardness | 0.0041 | hard | 0.0265 | ||
| 2A11(LY11) | soft | 0.0064 | QA15 | hard | 0.0047 |
| hard | 0.0175 | 08, 10, Q215 | 0.0032 | ||
| 2A12(LY12) | soft | 0.007 | 20, Q235 | 0.005 | |
| hard | 0.026 | 30, 35, Q255 | 0.0068 | ||
| T1, T2, T3 | soft | 0.0019 | 50 | 0.015 | |
| hard | 0.0088 | T8 | annealing | 0.0076 | |
| H62 | soft | 0.0033 | cold hardness | ||
| semi-hard | 0.008 | ICr18N9Ti | annealing | 0.0044 | |
| hard | 0.015 | cold hardness | 0.018 | ||
| H68 | soft | 0.0026 | 65Mn | annealing | 0.0076 |
| hard | 0.0148 | cold hardness | 0.015 | ||
| QSn6.5-0.1 | hard | 0.015 | 60Si2MnA | annealing | 0.125 |
If the necessary materials are not available above, you can also refer to the table below to find the modulus of elasticity and yield strength of the material, and then substitute them into the formula above for calculation.
| Material name | Material grade | Material Status | Ultimate Strength | Rate of elongation(%) | Yield strength/MPa | Elastic modulusE/MPa | |
| resisting shear/MPa | tensile/MPa | ||||||
| Carbon structural steel | 30 | Normalized | 440-580 | 550-730 | 14 | 308 | 22000 |
| 55 | 550 | ≥670 | 14 | 390 | – | ||
| 60 | 550 | ≥700 | 13 | 410 | 208000 | ||
| 65 | 600 | ≥730 | 12 | 420 | – | ||
| 70 | 600 | ≥760 | 11 | 430 | 210000 | ||
| Carbon structural steel | T7~T12 T7A-T12A | Annealed | 600 | 750 | 10 | – | – |
| T8A | Cold hardened | 600-950 | 750-1200 | – | – | – | |
| High quality carbon steel | 10Mn2 | Annealed | 320-460 | 400-580 | 22 | 230 | 211000 |
| 65M | 600 | 750 | 18 | 400 | 211000 | ||
| Alloy structural steel | 25CrMnSiA 25CrMnSi | Low-temperature annealed | 400-560 | 500-700 | 18 | 950 | – |
| 30CrMnSiA 30CrMnSi | 440-600 | 550-750 | 16 | 1450850 | – | ||
| High-quality spring steel | 60Si2Mn 60Si2MnA 65Si2WA | Low-temperature annealed | 720 | 900 | 10 | 1200 | 200000 |
| Cold hardened | 640-960 | 800-1200 | 10 | 14001600 | – | ||
| Stainless steel | 1Cr13 | Annealed | 320-380 | 400-170 | 21 | 420 | 210000 |
| 2Cr13 | 320-400 | 400~500 | 20 | 450 | 210000 | ||
| 3Cr13 | 400-480 | 500~600 | 18 | 480 | 210000 | ||
| 4Cr13 | 400-480 | 500-500 | 15 | 500 | 210000 | ||
| 1Cr18Ni9 2Cr18Ni9 | Heat treated | 460~520 | 580-610 | 35 | 200 | 200000 | |
| Cold-hardened | 800-880 | 100-1100 | 38 | 220 | 200000 | ||
| 1Cr18Ni9Ti | Heat treated softened | 430~550 | 54-700 | 40 | 240 | 200000 | |
It is best to establish a commonly used material database and obtain missing physical parameters from suppliers. If the parameters for elastic modulus and yield strength are correct, the bending and rebound of general spring terminals, appearance parts, and profiles are more precise.