Air Bending Force Chart (Amada)
In this blog post, we'll explore the fascinating world of air bending and the force charts that make it possible. Join us as we delve into the science behind this…
Have you ever wondered how to accurately calculate the bending force required for sheet metal fabrication? In this insightful blog post, we’ll explore the intricacies of bending force calculation, drawing from the expertise of seasoned mechanical engineers. Discover the key factors that influence bending force and learn how to apply proven formulas to optimize your metal forming processes. Get ready to elevate your sheet metal bending knowledge to new heights!
At present, the formulas for calculating bending force that are widely used have been adopted from foreign sources without any information about their origin or scope of application.
This article presents a systematic analysis of the derivation process of the formula for calculating bending force, as well as the required parameters.
Furthermore, a new approach for calculating bending force is introduced to broaden its scope of application.
Calculating the bending force for sheet metal is essential for achieving accurate and efficient bending processes. Understanding these calculations helps in selecting appropriate tools and ensuring quality bends. This chapter provides an overview of the key concepts, tools, and practical tips for calculating bending force in sheet metal work.
Bending force calculations are crucial in sheet metal work to ensure that the correct amount of force is applied during the bending process. This prevents material wastage, tool damage, and ensures the desired bend quality. Accurate calculations help in selecting the right press brake and tooling, leading to efficient production and cost savings.
The bending force ( F ) required to bend a sheet metal can be calculated using the formula:
where:
The internal radius ( R ) of the bend is often approximated based on the die opening ( V ):
The die opening ( V ) is selected based on the sheet thickness ( S ):
The minimum flange length ensures the material does not fall during the bending process and is calculated considering the die opening and bend angle.
In recent years, the press brake machine has gained widespread use across various industries and has expanded its processing capabilities.
Despite its popularity, there has been a lack of systematic discussion on the calculation of bending force.
Currently, there are approximately two types of bending force calculation formulas recommended by the product manuals of different press brake manufacturers.
In the formula:
The recommended formula for calculating the bending force by the manufacturer is based on a previously mentioned formula.
Both of these formulas have been taken from various product brochures, however, there is no proof of their accuracy.
Related calculator: Press Brake Tonnage Calculator
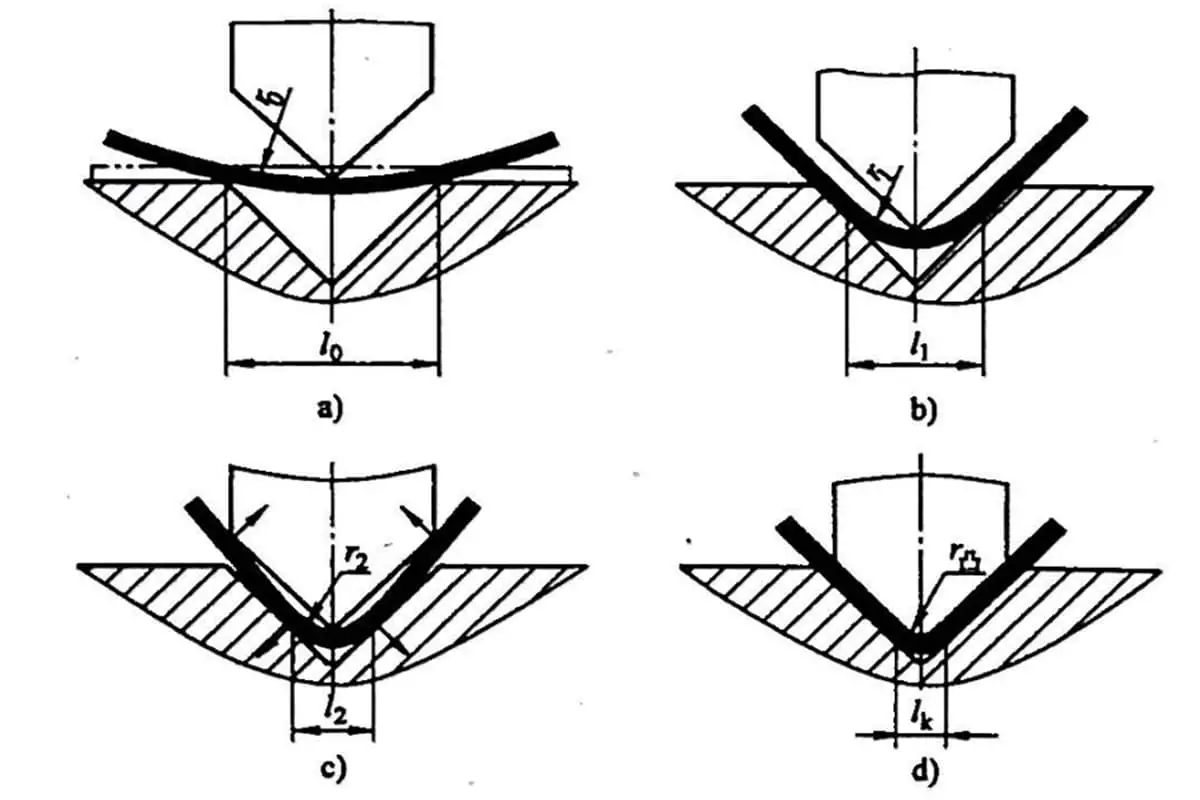
Figure 1 is a schematic representation of the bending process of a sheet.
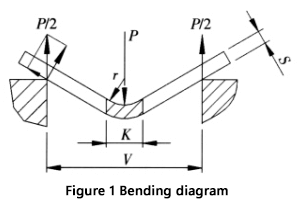
The calculation of the bending force and its parameters are explained as follows:
The recommended width of the lower die opening (V) for free bending is 8 to 10 times the sheet thickness (S), with a width-to-thickness ratio of V/S = 9.
Press brake manufacturers provide the values of the die width (V) and the inner radius (r) of the bent workpiece in their bending force parameter table. The radius-to-width ratio is usually r = (0.16 to 0.17) V, and in this case, the value of 0.16 is used.
During the bending process, the material in the deformation zone undergoes significant plastic deformation, causing it to bend around the centerline.
In some instances, small cracks may appear on the outer surface of the curved area.
The stress in the deformation zone, except near the central layer, is close to the material’s tensile strength, with the upper part of the neutral layer being compressed and the lower part being in tension.
Figure 2 illustrates the cross-section and corresponding stress diagram in the deformation zone.
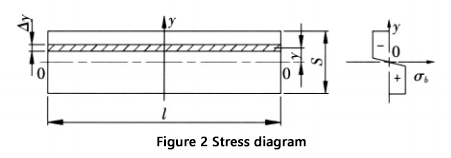
The bending moment at the section of the deformation zone is:
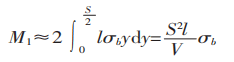
The bending moment produced by the bending force in the deformation zone is depicted in Figure 1.
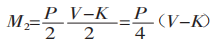
From M1 = M2, we get:
When bending a sheet with a universal mold on a bending machine, as shown in Figure 3, most sheets are bent to 90°. In this case, K is:
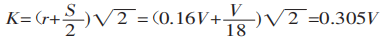
By substituting K into equation (1), we obtain:
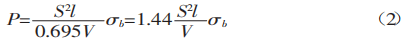
The tensile strength of ordinary materials, σb, is 450 N/mm². This value can be used in formula (2) to calculate the result.
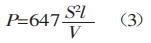
The formula for calculating the bending force obtained here is in agreement with the information provided in foreign brochures.
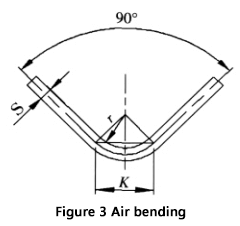
The variables in the formula are:
As can be seen from the derivation process, when using formulas (2) or (3) to calculate the bending force, it is important to ensure that two additional conditions are met: the ratio of width to thickness (V/S) must be equal to 9, and the ratio of radius to width must be equal to 0.16.
If these conditions are not satisfied, significant errors may result.
The calculation of bending force can be complicated when it is not possible to meet the two additional requirements (width-to-thickness ratio V/S = 9 and radius-to-width ratio = 0.16) due to design or process limitations.
In such situations, it is advisable to follow these steps:
These steps will provide a more precise and dependable result compared to using the commonly used formula. An example to illustrate this process is shown in Figure 4.
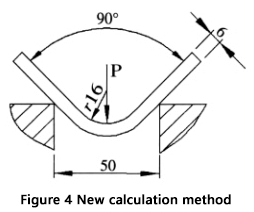
Given: Sheet thickness (S) = 6mm, Sheet length (l) = 4m, Bending radius (r) = 16mm, Lower die opening width (V) = 50mm, and Material tensile strength (σb) = 450N/mm².
Question: How can we calculate the bending force required for air bending?
Here are the steps:
First, calculate the ratio of width to thickness and the ratio of radius to width:
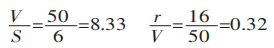
Then calculate the projected width of the deformation area:
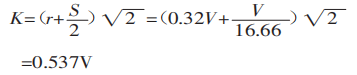
Finally, use formula (1) to calculate the bending force:
If the usually recommended formula is used to calculate the bending force:
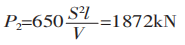
It can be inferred from P1/P2 = 1.5 that the difference between P1 and P2 is 1.5 times.
The reason for this discrepancy is because in this example, the bending radius is relatively large, which results in an increased deformed area and therefore requires a greater bending force.
The ratio of radius to width in this example is 0.32, which surpasses the previously mentioned criteria.
Using the standard formula to calculate the bending force is not suitable for this scenario. The advantages of using the new method for calculation can be observed in this example.
Additionally, there is an online calculator available to calculate the bending force using the new method.
Tensile Strength Table
| Material | Tensile strength | ||
|---|---|---|---|
| American | European | China | N/mm² |
| 6061 Aluminum | Alu50 | LD30 | 290 |
| 5052 Aluminum | Alu35 | LF2 | 303 |
| 1010 Mild steel | DC01 | 10/10F | 366 |
| A 536 -80 G 60-40-18 | GGG-40 | QT400-18 | 400 |
| A 351 G CF 8 | G-X 6CrNi 18 9 | Q235 | 450 |
| A 572 G50 | S 355 MC | Q345 | 550 |
| 304 Stainless | Inox V2A | 0Cr18Ni9 | 586 |
| 316 Stainless | Inox V4A | 0Cr17Ni12Mo2 | 600 |
| 4140 Low alloy | 42 CrMo 4 | 42CrMo | 1000 |
The formulas for calculating coining parameters are different from air bending.
1. Width of the die vee:
V = sheet metal thickness × 5
2. The internal radius is determined by the punch tip, which should be chosen in accordance with the following formula:
Radius = sheet metal thickness × 0.43.
3. Force required for coining：
F(kn/m)=Thickness2×1.65×Tensile Strength (N/mm2)×4.5/Die Vee Width
4. The formula for calculating the minimum internal edge remains the same:
Minimum internal edge = Die vee × 0.67
Some tools need a particular force to yield the sheet metal and to manage springback in order to obtain the profile re-quired.
As an example we will consider joggle tools, which make two bends at once with a short distance between bend and counterbend.
As these tools make two bends at once, springback has to be canceled completely by coining.
The equation to calculate the necessary force is:
Joggle tools usually consist of an insert holder into which the joggle tools chosen in accordance with the joggle and the angle required are secured with grub- screws.
It is important to ask for technical advice from the manufacturer before purchasing, because these systems can only bend thin sheet metal, a maximum 2mm, but the maximum thickness will depend on the type of insert and it could be less than 2mm.
Below are answers to some frequently asked questions:
Tensile strength plays a crucial role in determining the bending force required for sheet metal. Tensile strength is the maximum stress a material can withstand while being stretched before it breaks. When sheet metal is bent, it experiences both tensile and compressive stresses. The outer surface of the bend is subjected to tensile stress, while the inner surface undergoes compressive stress.
The bending force required is directly influenced by the material’s tensile strength because higher tensile strength means the material can withstand greater stress without failing. This implies that for materials with higher tensile strengths, greater force is needed to achieve the same degree of bending compared to materials with lower tensile strengths.
To calculate the bending force, the bending stress, which is related to tensile strength, is considered. The bending stress formula
involves the bending moment (M), the distance from the neutral axis to the outermost fiber (c), and the moment of inertia of the cross-section (I). The bending moment is influenced by the applied force and the length of the lever arm. Ensuring that the bending stress does not exceed the material’s tensile strength is vital to prevent failure during bending.
In summary, higher tensile strength necessitates a higher bending force to bend sheet metal without causing it to fail, highlighting the importance of considering material properties in bending force calculations.
When calculating the bending force for sheet metal, several key factors need to be considered to ensure accurate and reliable results. The main factors influencing bending force calculations include:
Material properties play a crucial role, particularly tensile strength and yield strength. Tensile strength is the maximum stress a material can withstand before failing, with different materials having varying strengths. For example, aluminum has a tensile strength of 200-300 N/mm², mild steel (Q235) ranges from 370-500 N/mm², and stainless steel is around 650-700 N/mm². Yield strength determines the stress at which the material begins to deform plastically, with higher yield strength requiring more bending force.
Material thickness significantly impacts the bending force, as thicker materials resist deformation more, necessitating greater force. The calculation formulas typically include material thickness as a key variable.
Bending radius and angle are also important. The inside bend radius, or the radius of the bend, influences the force required, with smaller radii needing more force due to increased stress concentration. The bend angle affects the force needed, with most calculations based on a 90-degree bend but requiring adjustments for different angles.
Tooling and die configuration, specifically the lower die vee opening, affects the bending force. The width of the lower die channel in the bending machine is related to the material thickness, and precision bending may require a narrower V opening for thinner materials.
The K-factor and the position of the neutral axis also influence the bending process. The K-factor, while more directly related to calculating bend allowances and deductions, affects the overall bending behavior and force required by determining where the material is neither compressed nor stretched.
Lastly, the bending force calculation typically involves a formula that incorporates the material’s tensile strength, thickness, and length of the bend. For example, a simplified formula for low-carbon steel is:
where ( t ) is the thickness in mm and ( l ) is the length in meters, with the result in tons.
By considering these factors, you can accurately calculate the bending force required for sheet metal bending operations, ensuring the press brake machine is set correctly to achieve the desired bend without causing material failure or excessive deformation.
To determine the appropriate settings for a press brake machine, you need to consider several key factors to ensure precise and efficient bending of sheet metal. First, identify the material type and thickness, as these significantly influence the required bending force. Different metals, such as stainless steel and aluminum, have varying strengths and elasticity, which affect the force needed for bending.
Next, calculate the required tonnage using the formula ( P=650S²L/V ), where ( P ) is the bending force, ( S ) is the thickness of the metal plate, and ( V ) is the opening of the bottom die. Take into account the bend length and bend angle, as sharper angles require higher tonnage. Ensure proper material support and alignment to prevent deflection and inaccuracies.
Different bending methods, such as air bending, bottom bending, and coining, require different tonnages, with air bending being the baseline and coining requiring about eight times the air bend tonnage. Check that the tonnage does not exceed the central tonnage limit of the press brake to avoid overloading and potential damage.
Select appropriate tooling that matches the material and bending requirements, ensuring the tooling is properly aligned and installed. Calibrate the machine by inspecting and cleaning it, adjusting the backgauge, and calibrating the ram position and angle. Program the press brake according to the calculated tonnage and bending parameters, and perform a test run on scrap material to fine-tune the settings and ensure the desired results.
By following these steps, you can accurately determine the appropriate settings for your press brake machine, leading to precise and efficient sheet metal bending operations.
The formulas and steps provided for calculating the bending force are suitable not only for angular bending of a sheet, but also for arc-shaped bending (which technically should be referred to as angle bending with a large bending radius).
It is crucial to keep in mind that forming an arc shape requires a unique mold design.
When projecting the deformation area, the calculation must be based on the process parameters established during the process, which cannot be determined through a single formula.
At a specific iron tower factory, we successfully bent a cylinder with a wall thickness of 12mm, a diameter of 800mm, and a length of 16m using a 28000kN press brake machine and a circular mold.
The method outlined in this article was utilized to determine the bending force and produced satisfactory results when designing a mold for an arc shape.
Further reading:
As the founder of MachineMFG, I have dedicated over a decade of my career to the metalworking industry. My extensive experience has allowed me to become an expert in the fields of sheet metal fabrication, machining, mechanical engineering, and machine tools for metals. I am constantly thinking, reading, and writing about these subjects, constantly striving to stay at the forefront of my field. Let my knowledge and expertise be an asset to your business.
EN 
AR 
DE 
ES 
FR 
IT 
JA 
NL 
PT 
PT_BR 
RU 
KO